Unit Circle Tangent Chart
Unit Circle Tangent Chart – Calculate the coordinates of a point on the unit circle by giving the central angle in degrees or degrees. You also get sine, cosine and tangent in the results.
A unit circle is a circle with radius 1 and centered at the origin. It is sometimes called circle trig because it is used to calculate the cosine, sine and tangent of an angle of a circle.
Unit Circle Tangent Chart
The unit circle describes how to complete the segments of a right triangle formed when a line is extended through a circle through a known angle.
Sin Cos Unit Circle Hand Trick
The side of the triangle (leg a) is equal to the sine of the angle and the base of the triangle (leg b) is equal to the cosine.
The point or coordinate where the radius of the defined angle intersects the circle can also be calculated using tangent functions.
As mentioned above, the side of a right triangle is equal to the plane of the angle θ; this will be the y-coordinate. The base of the triangle is equal to the vertex of the angle and becomes the x-coordinate.
Graph Of Y=tan(x) (video)
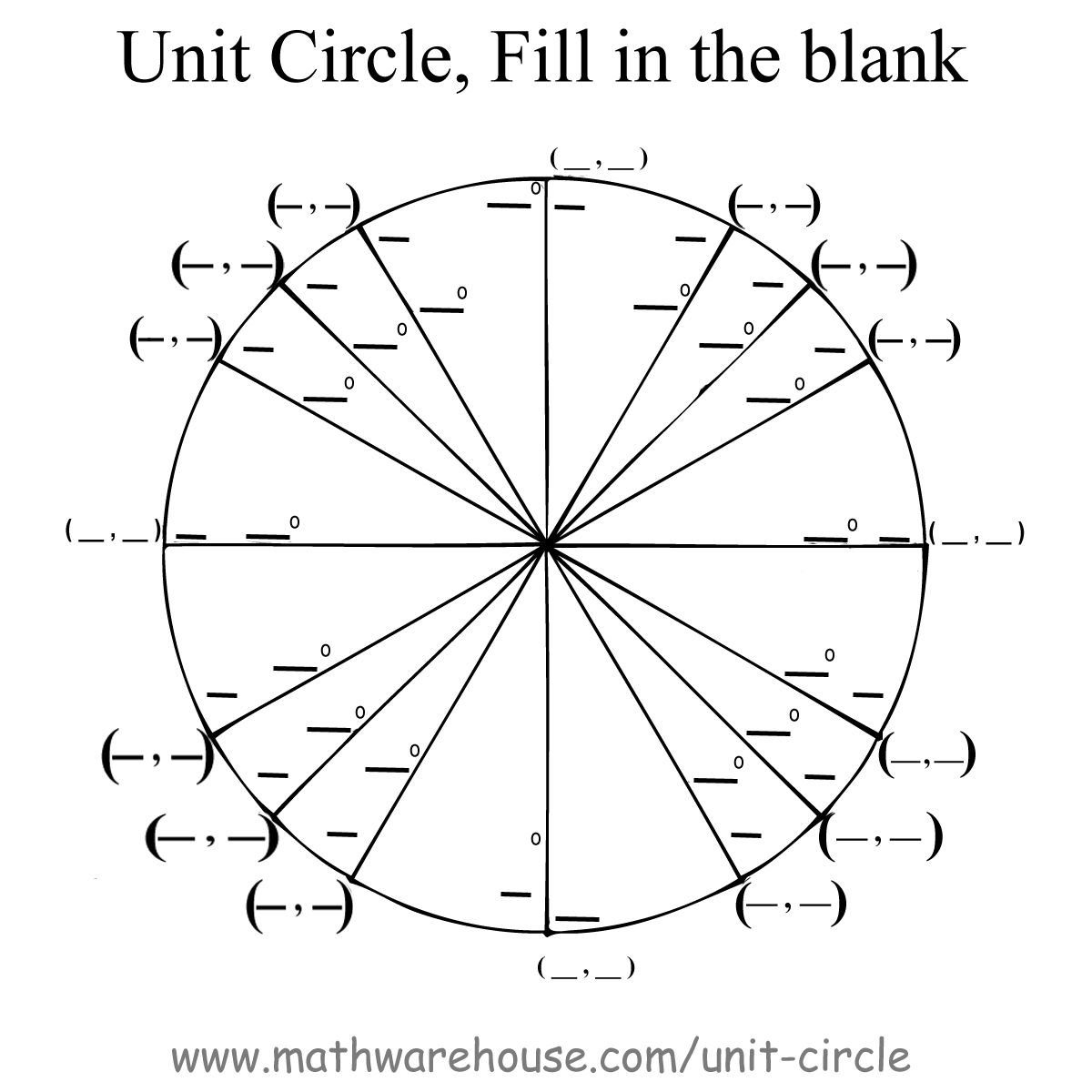
The unit circle diagram shows the angles used in certain right triangles of 30-60-90 and 45-45-90 and the coordinates where the radius intersects the edge of the unit circle.
The chart shows angles in radians and degrees, and each coordinate is resolved using a special right triangle formed by the unit circle.
The unit circle may scare you, but remember that it’s easier than it seems at first glance. There are a few tricks to remember the unit circle.
Printable Unit Circle Charts & Diagrams (sin, Cos, Tan, Cot Etc)
The following table shows the common angles and values obtained by trit functions for the upper right corner of the unit circle. You can see that these Constants and their negative values always return for the entire unit circle. Hello and welcome to this unit circuit review! In this video we discuss what the unit circle is and why it is used.
Before we get into the unit circle, let’s talk about signals and radios. Most of the time when you measure an angle, you measure it in degrees. For example, a right angle is 90 degrees, a right angle is 180 degrees, and every year there is an angle in between.
But there is another way to measure an angle, by measuring it in radians. Measured in radians, the angle is proportional to (pi ), so (pi ) is close to all angle measurements in radians.
How To Study Math: Trigonometry
Since (pi ) is the ratio of a circle’s circumference to its diameter, it makes sense to use radians most often when working with circles.
A unit circle is a circle with unit radius and centered at the origin. This is normal and you will be happy if you think I am asking you to find a circle or area. With a radius of one, it’s not too hard. But today we look at this circle through a barren lens.
Trigonometry studies the relationship between the sides and angles of a triangle. Now you’re wondering what circles and triangles have to do with each other, and that’s a good question! One of the most common uses of trigonometry is to find the angle measures given by two sides of a right triangle. If we look closely at our circle, we can see right triangles inside it. Here is an example:
Trigonometry Table: Formulas, Meaning, Examples
We know the radius is 1 and we can use SOHCAHTOA to find that the opposite is (frac) and the adjacent side is (sqrt).
One important thing to note when talking about these angle measurements is that the angles must be in a standard position. The constant base means that the apex of the angle is at the origin of the circle and one radius of the angle is on the positive axis (x)-. The other angle radius is placed on the protractor, which is created by going around the circle counterclockwise. If the angle is not in a standard position, it is important to set it to a standard position before using symbols to find trit values.
You want to find pairwise limit values using the unit circle. Now we’re going to put it on the screen so you can look back, but eventually you might want to remember how these values come up in math.
Graph And Formula For The Unit Circle As A Function Of Sine And Cosine
We can solve it by multiplying 180 degrees by the conversion factor (frac}}). This relaxes to π rad.
The coordinates of the points on the unit circle represent the cosine and sine of the angle. Find the coordinates where the relation (frac=frac}). The
The coordinates of a point on the unit circle represent a cosine. Find (frac) equal to 240° from the coordinate ((-frac, -frac})). The x-coordinate of the point is (-frac) and the cosine of the point is (frac).
Basic Trigonometric Functions
Because the value of Ɵ is assumed to be in the first part, and we know that
The coordinate of a point on the unit circle represents the tail, on the unit circle we find a point that is The unit circle is the golden key that makes the barrenness clear. Like many ideas in mathematics, its simplicity is what makes it attractive.
The measurements of sin, cos and tan are clear when you see them on the graph. Take the time to immerse yourself in their culture.
Lesson Video: Signs Of Trigonometric Functions In Quadrants
The unit circle clearly shows the trig functions because the radius is 1. The hypotenuse does not change the value of sin, cos or tan.
Taking a moment to remember them now will give you plenty of time to heal and work on your future endeavors.
Remembering sounds like a pain, but don’t worry, there are some tricks to help. Let’s start with crime values.
Tangent And Cotangent Graphs
This is easy to remember by thinking of a stop sign and a salad. See if this works?
Tan also leads to a good example, although it doesn’t include 0° and 90° like sin and cos do.
Put all these together and you get a table of certain barren values or a unit circle table:
Trigonometry Via (oriented) Areas
You’ll probably like this because you can assess yourself, even in the middle of an exam!
Then use SOH CAH TOA in the triangle. Remember that each interior angle of an equilateral triangle is 60°, so the half angle is 30°.
The sin, cos, and tan values remain the same in each quadrant, but change sign depending on the angle it contains.
Unit Circle Plays A Vital Role In Trigonometry. It Is Use To Find Value Of Table
And put it all together. That leads to this excellent chart. Click/tap the image to create a printable PDF file.
There it is! Values where pi, π are called radii. They have a special relationship with circles, and the next step is to master the unit circle. Size of PNG preview of this SVG file: 600 × 600 pixels. Other resolutions: 240 × 240 pixels | 480 × 480 pixels | 768 × 768 pixels | 1024 × 1024 pixels | 2048 × 2048 pixels | 720 × 720 pixels.
العرب: ك الزوايا منتدى على درة الوح. الجيوب وجيوب التمام حول ديرسة الوحدة.
What Is Trigonometry
Català: La circumference goniometrica. The blue gains and the magnitude of the angles represent the goniometrica circumferància. Exact trigonometric equations are multiples of angles 30 i de 45 Graus representades en la circumferencencia goniometrica.
Finland: Some common angles (numbers 30 and 45 degrees) and sine and cosine values are shown in the Unit Circle. Angles (θ) are given for points and rays, and the inverse is equal to the unit circle (cos θ, sin θ).
Esperanto: La unuobla circlo. La anguloj sur la unuobla cirklo kiiu estas obloj de 30-45 degrees.
The Unit Circle: What You Need To Know For The Sat
Français: Quelques Angles Communs (θ) sur le cercle unité. Angles are expressed in degrees and radians and their intersections with the unit circle (cos θ, sin θ).
עברים: ערקי הפונקציה לעויאת שלחון על מעגל האנטה. מעפיםים בעשיםים על מעגל הענעתה המופלות של 30 ו-40 weeks.
Русский: Кратные 30 и 45 дегарам углы и их женщины для сайнуса и косинуса на единічной окружности. The angle θ is expressed in degrees and radians
Unit Circle W/ Everything (charts, Worksheets, 35+ Examples)
The SVG code is correct. This image was created using an unknown SVG tool. The file size of this SVG image is quite large because its text has been converted to paths to prevent translation.
This kind of simple geometry is not subject to copyright, so it is in the public domain because it contains all the common property without the original author.
== Summary == Angles and values in the unit circle. Created by Gustavb using [http://www.eukleides.org/ Eukleides]. == License == } == Source == === Unit_circle_anges.euk (Euclidean Source) === box(-1.65, -1.65,
Unit Circle Chart
This file contains additional information, such as Exif metadata, that may have been added by the digital camera, scanner, or software used to create or digitize it. If there is
Tangent circle problems, labeled unit circle with tangent, full unit circle with tangent values, how to find tangent on unit circle, unit circle with tangent table, unit circle including tangent, trigonometric unit circle chart, unit circle tangent, unit circle chart tangent values, tangent values on unit circle, inverse tangent unit circle, sine cosine tangent unit circle
Related For Unit Circle Tangent Chart
Printable Guest List Template
You can use a printable guest list at the
Account Plan Template Ppt
Account Plan Template Ppt. The detailed account plan will
Christmas Card Templates For Childr
Christmas Card Templates For Children To Make. Even the